By Vishal Kumar
Since March of 2017, I have been enriching my understanding of design through mathematics — specifically, ancient geometry. As you scroll down, I hope my findings will be enriching for you too!
I provide three demonstrations to explain how theorems from ancient mathematics can help you improve your design skills.
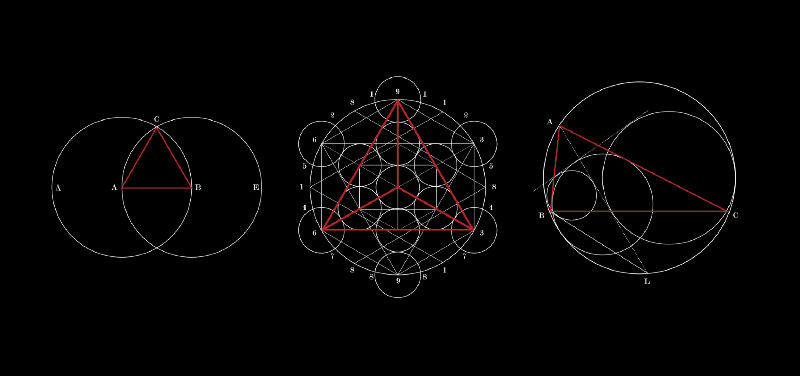
To begin with, theorems from ancient mathematics can be simple, beautiful, and artistic. Take, for example, an equilateral triangle. You only need two circles of the same size to make a perfect equilateral triangle.
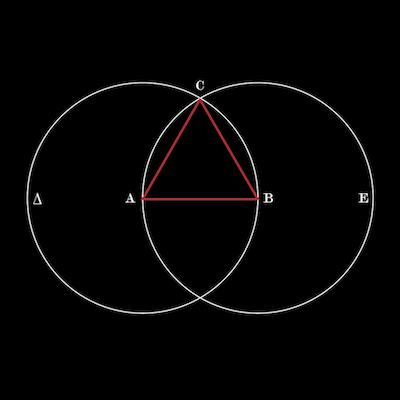
Draw the circle on the left. Then draw a straight line from the middle of that circle (A) to the end (B). Draw a circle exactly the same size on the right so that it passes through A. Then draw two straight lines from A and B to the intersection of the two circles (C).
Easy, right? Didn’t even need a calculator. It’s incredible to think that the red triangle above has sides that are all of the same length, with all inside angles being 60° — and we didn’t even need any numbers to make it!
“I never knew math could be so simple.”
This is the first theorem of a book entitled The Elements, written over 2,300 years ago by an Ancient Greek mathematician, Euclid. It has been estimated to be second only to the Bible in terms of the number of editions published since mechanical printing was invented in the 15th Century.
The Elements was so influential that Abraham Lincoln had a copy on his desk at all times. (Cool story).
Hold on, it gets better. Let’s take it up a level.
There are many mysteries to an equilateral triangle. The same red equilateral triangle you saw above can be used to generate a range of other shapes and forms.
Below we see that the equilateral triangle can help draw a circle, hexagon, rectangle, and a whole range of other polygons. See how many you can find.
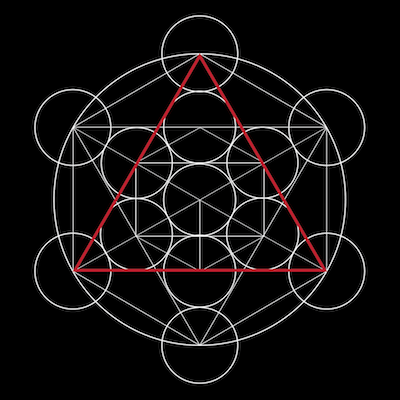
Throughout history the equilateral triangle has been fundamental to human civilization, society and religion.
In his 2010 paper, Mysteries of the Equilateral Triangle, mathematician Brian McCartin explains how the shape has helped with a broad range of designs — from map-building, to problem-solving, to creating works of art and making religious symbols and relics.
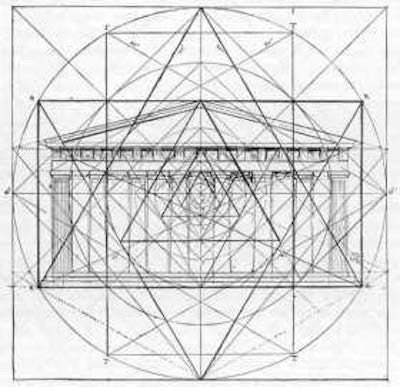
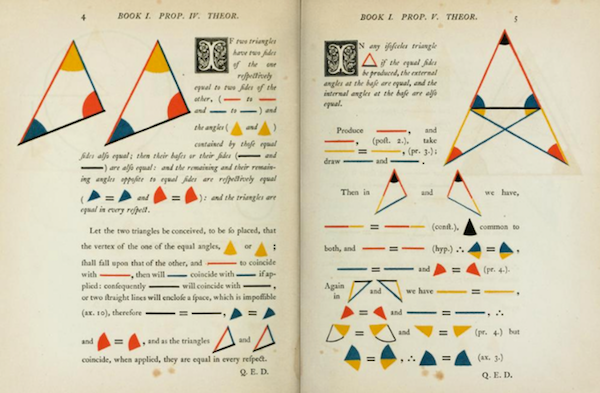
Let’s look at architecture in Ancient Greece. The figure below (left) is very similar to my one above. It shows the facade of the Parthenon, built in 432 BC, together with superimposed concentric equilateral triangles, each successive triangle diminished in size by one-half. This diagram helps visualize the perfect and well-crafted proportions of the Parthenon.
It’s clear that the Greeks knew about the importance of geometry.
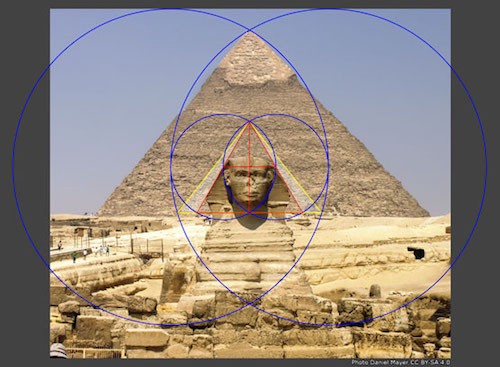
at Giza — Brian McCartin (2010)
Another, and older, common example of equilateral triangles used in architecture is the Pyramid Complex of Giza in Egypt. Each of the four triangular sides that form the pyramids are equilateral triangles. These are examples of the strength of the triangle in architecture as the pyramids have been standing for over 4,000 years.
Why is any of this important?
Taking a geometric approach to drawing basic, smooth shapes can set the foundations for improving one’s design skills.
A geometric approach allows you to organize and arrange your space in a much easier way — whether your space is a computer or mobile screen, your notebook, or even a post-it note.
Look at how Apple designed their logo. Inkbot Design challenges Apple’s logo, dissecting it and asking whether their logo was designed using the Golden Ratio.
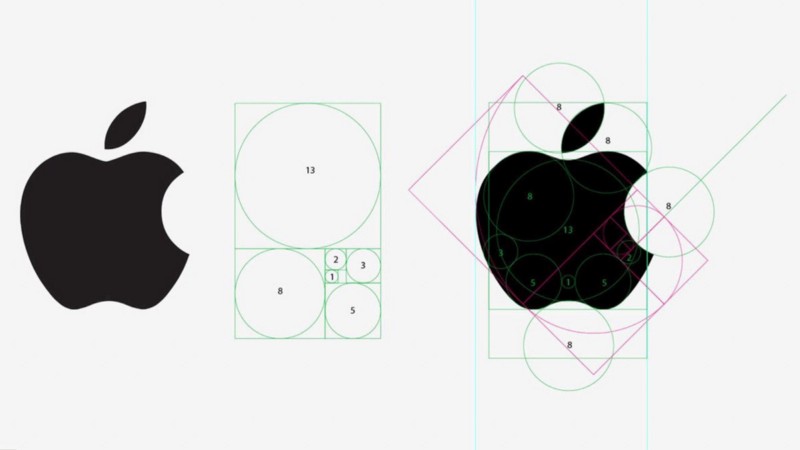
Related story: Jinju Jang also explains how she used mathematics and geometry to improve her design skills.
So far the equilateral triangle has been the star of my story, but it is only the first of many interesting characters and protagonists.
Euclidean geometry is the classic geometry we learn at school to make shapes with ‘smooth’ edges, such as a triangle or circle. Euclidean geometry proceeds logically from axioms, to theorems and then to three-dimensional space.
If you add in a ‘time’ dimension you get Newtonian physics, forming a single space-time continuum.
[Kroneker Wallis, a minimalist production team in Barcelona, have even created contemporary designed books that explain precisely this in more detail! This also validates my point about ancient mathematics being important for design today — so, support them on Kickstarter!]

“I never knew math could be so artistic!”
However, there are adaptations of geometry to make infinitely complex shapes with ‘rough’ edges and across multiple dimensions in addition to space and time: design materials based on biological or environmental properties, for example.
Enter fractal geometry.
Fractal geometry is used across the natural sciences — mathematics, physics, chemistry and biology — but more recently, for building and urban design. For example, Neri Oxman at the MIT Media lab computationally simulates fractal forms from nature to design and generate new materials and buildings (see below).
Moreover, Michael Batty from the Centre for Advanced Spatial Analysis at the Bartlett, UCL, explains that fractal geometry has much to do with the way cities evolve. His research computationally simulates the evolutionary process to suggest “good” urban design as opposed to “bad” urban design.
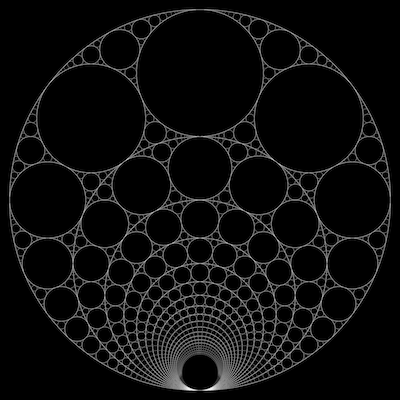
Fractal geometry is a field of mathematics born in the 1970s and mainly developed by Benoit Mandelbrot. It can lead to self-similar forms across different scales, in this way evoking natural growth and design — the beautiful image below is a nested Apollonian gasket.
The theory of fractals can also be linked to complexity theory and chaos theory — please look at the bottom left of Dominic Walliman’s Map of Mathematics poster.
Right, I promised that this post was not going to get boring, so I am going to stop here. (I hope it has been enriching!)
Conclusion
I would like to highlight two major points made in this article:
- Simple ideas from geometry and mathematics can be helpful and profound for those interested in all spectrums of design. Matej Latin describes how he used geometry to get a pixel perfect User Interface element for his project.
- It is possible to use more complex strands of geometry to create much more interesting shapes. For example, generating designs similar to natural forms.
I will continue to explore and explain how mathematics can help us understand design at a much deeper level. This first post was mainly about geometry, but I hope to also look at other fundamental ideas from algebra, calculus and trigonometry.
Thank you for reading!
Before you leave…
If you found this article helpful, hit the? button below and share the article on Facebook, Twitter or LinkedIn so that everyone can benefit from it too.
See more of Vishal’s work on Instagram @vishalkumar.london or on his website.